The objective of this experiment is to determine the mass of a spinning disk by measuring how quickly a hanging mass wrapped around its axle accelerates due to gravity.

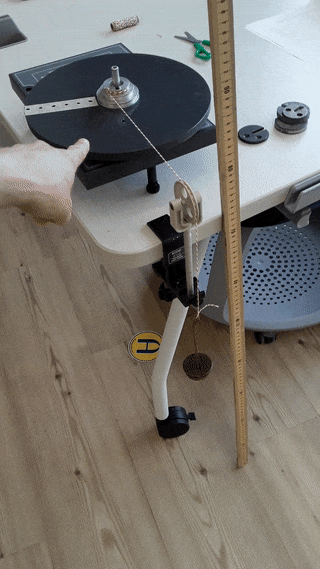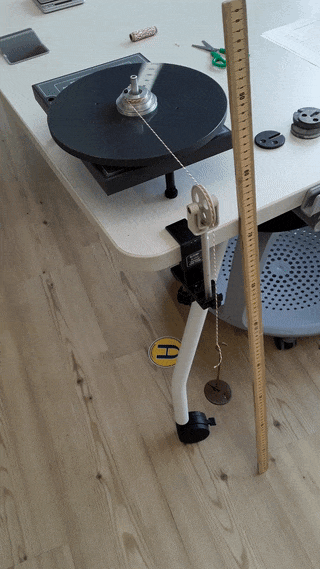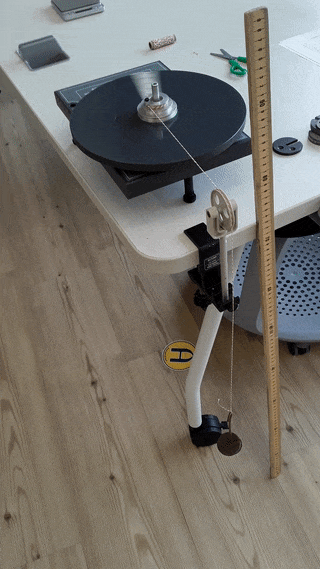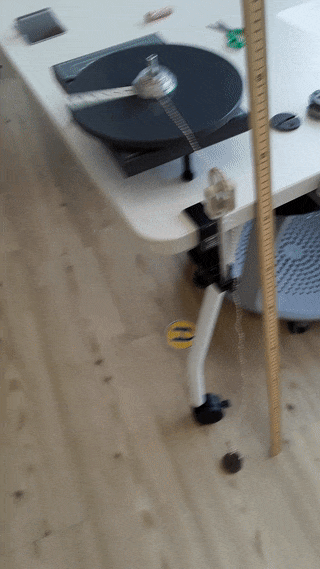
Resources

• Rotational Motion Apparatus
• Masses and hook attached to string
• Pulley
• Meter stick
• Time measuring device (e.g. phone, online-stopwatch.com)

Background

Rotational kinematic quantities have many analogies to linear ones. Angular acceleration \alpha, for example, is simply linear acceleration a divided by the radius r of circular motion.

\alpha = a/r

In the same way, torque, defined as \vec{\tau} = \vec{r} \times \vec{F} has a rotational analog to Newton’s second law \Sigma \ F=ma:

\Sigma \ \tau = I\alpha

where I is the called the moment of inertia, whose value depends on the distribution of mass in the rotating object with respect to its rotation axis. The moment of inertia of a point mass moving in a circle of radius is simply I=mr^2. The moments of inertia of many geometric forms can be calculated and tabulated by forming an integral that computes the sum of many point masses. The moment of inertia of a disk of mass M and radius R is:

I = \tfrac{1}{2}MR^2

In this experiment, the only torque acting on the pulley is the tension force at radius r from the mass as it is pulled down by gravity. The angular acceleration of the pulley can be calculated directly from the downward acceleration of the mass, and also predicted from the rotational form of Newton’s second law.

Method

1. Wrap the string of the hanging mass around the axle pulley of the disk on the Rotational Motion Apparatus and drape the string over the pulley clamped to the table edge.
2. Release the mass. From the initial height and time it takes to reach the ground, determine the acceleration, and thus the tension force in the string.
3. From the radius of the axle pulley, calculate the torque acting upon the spinning disk.
4. From the applied torque, disk radius, and angular acceleration, calculate the disk mass.

Analysis Questions

1. Explain your calculation of the disk mass step-by-step, and give the results leading to your answer.
2. Propagate the uncertainty in your measurements to your result.
3. Compare your calculated disk mass to that using the large scale at the front of the room. Do the values agree? Describe what specific effects might produce a systematic error in your result.