Tasks

1. Project images on a screen using a diverging lens and determine its focal length.
2. Build a very simple optical instrument: a Galilean telescope.

Resources

• Optical bench
• Light source
• Converging and diverging lenses and mounts
• Mounted white screen
• Ruler and/or meter stick

Background

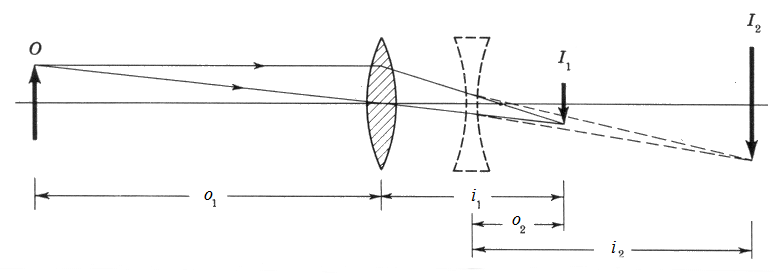
A diverging lens cannot produce a focused image on a screen of a distant object by itself, because the parallel rays from the object will only be spread apart further. However, it can produce a focused image with the help of another lens that makes those parallel rays into converging rays first.
Hint: show/hide

An object located a distance o from a lens of focal length f will produce an image at a distance i from the lens, according to the equation \frac{1}{o}+\frac{1}{i}=\frac{1}{f}

In this formula, o always has a positive value. Negative values of i give the position of a virtual image formed on the same side of the lens as the object, as shown in the diagram.

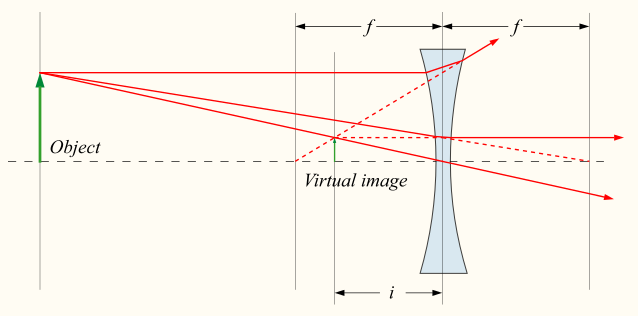
The apparent magnification of an object describes how much larger the image appears than the original object. It can be shown geometrically that this is related to the image and object distances. Negative magnifications mean an inverted image. m=-\frac{i}{o}

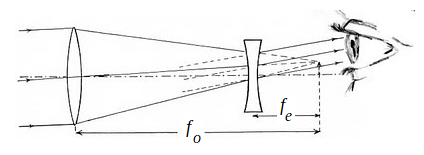
A simple optical instrument is the Galilean telescope. The purpose of a telescope is to focus and magnify parallel light rays from distant objects (e.g. stars) and return those focused rays to parallel so they can be easily viewed by the eye. A Galilean telescope uses a converging lens and a diverging lens as shown below. The magnification of a Galilean telescope is given by m=f_o/f_e, where f_e is the focal length of the eyepiece lens and f_o is the focal length of the objective.