Objectives

1. Determine the effective frictional force produced by a friction slider dragging behind a cart pulled by a hanging mass.
2. Measure the frictional force under many different applied forces and masses applied to the friction slider to find a value for the coefficient of kinetic friction \mu_k between the slider and track.
3. Determine the coefficient of static friction \mu_s between the slider and track.

Resources

• Flat dynamics cart track
• Cart with force sensor attached
• Motion Sensor
• Science Workshop interface and DataStudio software
  
  • Datastudio setup file: newtonslaw_motionsensor.ds
• Pulley and string
• Mass hanger and masses
• Friction slider and weights

Background

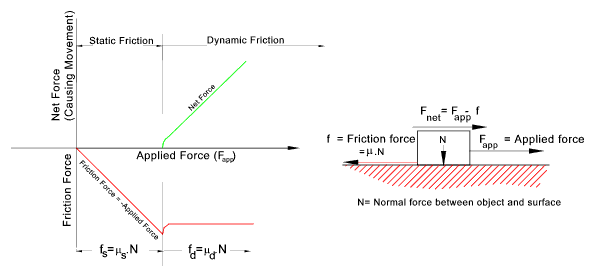
When surfaces in contact move relative to each other, the friction between the two surfaces converts kinetic energy into thermal energy. The details of how this happens are actually quite complex, and can be a combination of inter-surface adhesion, surface roughness, surface deformation, and surface contamination. However, the resulting force can be modeled with simple equations of static/kinetic friction. f_{s,max} = \mu_s N \\ f_k = \mu_k N where N is the normal force due to the object pressing against a surface, and \mu_k, \mu_s are the respective kinetic and static friction coefficients, the value of which are specific to the type of surfaces in contact as well as other environmental conditions. The static frictional force acts to oppose any applied force up to its maximum value, at which point the kinetic frictional force acts instead.

Method

Set up the experiment as shown in the image above. Release the cart and measure its acceleration and the applied tension for a range of hanging masses and masses added on to the friction slider. Compute the effective frictional force in each case, and calculate the kinetic friction coefficient.