Goals

1. Let a cart roll down an inclined track and show that the initial gravitational potential energy and final kinetic energy are equal.
2. Show that the final kinetic energy is the same for varying track inclines when releasing the cart from the same initial height.

Resources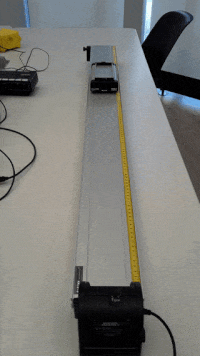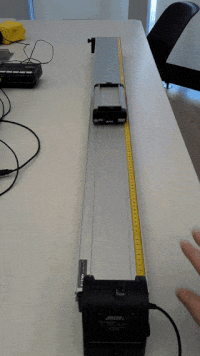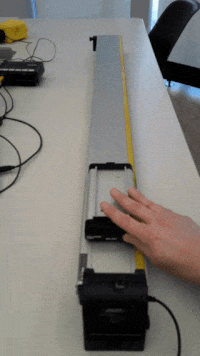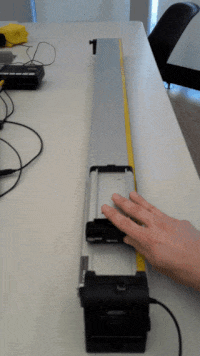

• Cart track with end bumper, adjustable legs
• Collision cart
• Motion sensor
• ScienceWorkshop interface and DataStudio software
  • Datastudio setup file

Background

Energy has the property that it is always conserved. It can be changed into other forms of energy, and sometimes this change is not easily reversible – as in the case of conversion to thermal energy due to friction – but energy can never be truly “lost”.

A cart of mass m at the top of a ramp has potential energy relative to the bottom of the ramp equal to PE_{grav}=mgh where h is the height of the cart above the end of the ramp. When the cart is released, assuming frictional forces are negligible, the cart will have kinetic energy KE=\tfrac{1}{2}mv^2 at the bottom of the ramp.

Method

Set up the track, motion sensor, and cart as shown in the image above. Release the cart and record its velocity and position vs. time. The setup file is configured so that DataStudio will stop automatically when the cart is within 20cm from the motion sensor. Measure the height of the cart at the initial and final positions. Calculate the change in potential and kinetic energy and compare the two values.

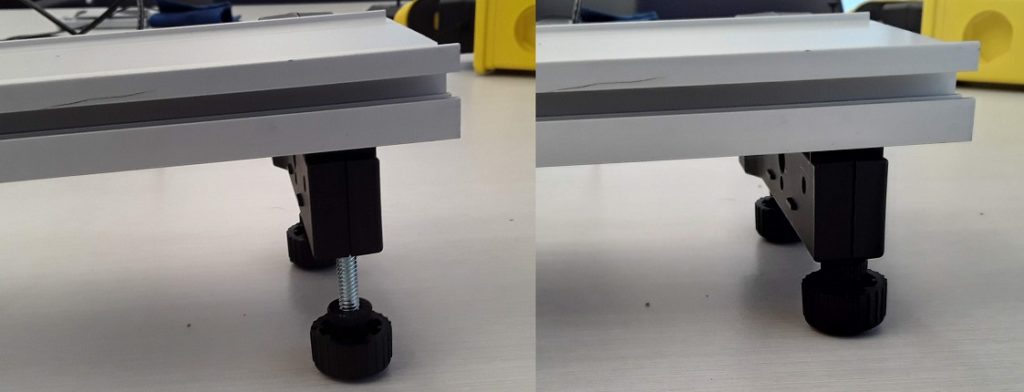
To adjust the height of the ramp, the legs can be extended as shown. Release the cart with different track inclines but the same initial height, and show that the final kinetic energy is the same.

Analysis Questions

1. Are the final velocities equal within your uncertainty?
2. Do you see any systematic trends in the data with different track inclines? If so, how do you explain them?