By building a simple capacitor, you can test some of the basic assumptions about charge, electric fields, and voltage.

Objectives

1. Make a simple parallel-plate capacitor
2. Measure the dielectric constant of a plastic container lid.

Resources

• Inductance/capacitance (LC) meter
• Metal foil tape
• Plastic container lid
• Wires with alligator clips
• Ruler, calipers
• Scissors

Background

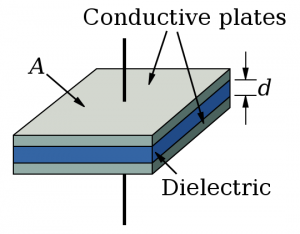A capacitor consists of two conductors separated by air or some other non-conducting material (dielectric). When a voltage is applied between the conductors (plates), an equal charge of opposite sign is accumulated on each plate, effectively storing energy in the electric field between the plates. The ratio of the charge stored to the applied voltage is the capacitance, with units of Farads (\mathrm{F = 1 Coulomb/Volt}).

A very simple form of capacitor involves two flat plates of equal area A separated by a constant distance d, which gives a capacitance of C=\kappa\varepsilon_0 \frac{A}{d}

The dielectric constant, \kappa, is a unitless value specific to the type of material used. It is 1 for vacuum and very close to 1 for air.

Guideposts/Hints

• Hint 1:
  The internet has many examples of instructions that describe how to build a simple capacitor using the provided materials.
• Hint 2:
  You will want to look up the dielectric constant for the container lid to compare it to your measurement. The resin identification code imprinted in the plastic can help you identify what it is made of.
• Hint 3:
  Don’t forget to estimate uncertainties in your measurements! You will need these to assess/justify the quality of your conclusions.
• Hint 4:
  Measuring the thickness of oddly-shaped objects with calipers: