We usually imagine electric fields as invisible lines in the air, but they can exist inside materials as well. In this exercise you will use a 9-Volt battery to produce electric fields in sheets of paper with shapes drawn on them in conductive paint.

Objectives

1. Draw a representation of the electric fields produced in two different sheets of paper with different conductive patterns.
2. Measure the approximate magnitude of the electric field at at least three different points in each pattern, and show that it changes in the way that is expected based on the shapes of the conductive patterns.

Resources

• Voltmeter
  • Video tutorial
• 9-Volt battery
• Wires with alligator clips and metal pushpins
• Paper with conductive paint patterns (2)

The electric field gives the magnitude and direction of the electric force per unit charge at every point in space. Voltage (also confusingly referred to as electric potential) is the potential energy per unit charge due to the electric field at a given point. Thus, voltage has its greatest increase in the direction opposite to the electric field at a given point, and lines along which the voltage is constant (i.e. equipotential lines) will always be perpendicular to the electric field.

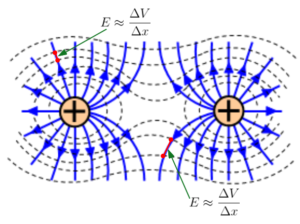
As long as the electric field is changing smoothly along a displacement \Delta s, the average electric field along the displacement is E_{avg}\approx \frac{\Delta V}{\Delta s}

Guideposts/Hints:

• Hint 1:
  You can set up an electric field in the paper with conductive patterns by connecting each of the terminals of the battery to a metal pushpin inserted through the paper and pressing against the conductive paint, as shown in the image.
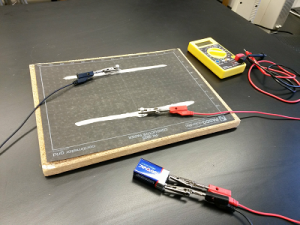
• Hint 2:
  A voltmeter measures the change in voltage between the two points where the probes are touched. So, you should keep one of the probes at a fixed point for all your measurements, otherwise your voltage measurements will have different reference points.
• Hint 3:
  To accomplish your first objective, you are trying to reproduce something like the figure shown below, only for a dipole and for two parallel plates instead of a single charge.
  
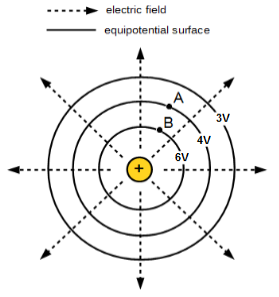
• Hint 4:
  You can’t directly calculate the expected electric field strength at every point on the paper because you don’t know the total charge. However, you can predict how the field strength will change relatively from point to point based on the formula for the electric field of a dipole or that for two parallel plates.